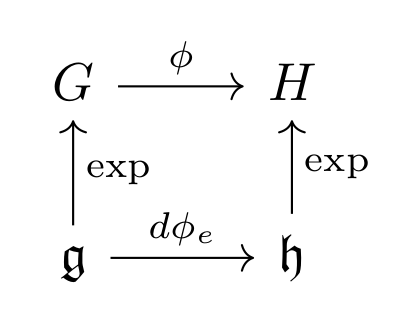
Overview: Lie theory brings together calculus, linear algebra, geometry, and more in a fascinating way. It is the theory of “continuous symmetries” such as the group of rotations of a sphere, with applications in physics, chemistry, and any subject that involves continuous motion. The Norwegian mathematician Sophus Lie created his theory largely to study differential equations, but soon applications of the subject were found in other areas of mathematics and science.
Matrix groups are often “curved” and have some nontrivial geometry. We shall analyze these groups by associating with them rather mysterious flat spaces known as Lie algebras and we shall also study them as they arise in applications.
Mathematical topics to be covered in MAT 472 include inner product spaces, geometry of complex numbers and quaternions, the orthogonal groups, Lie algebras, matrix exponentiation, and maximal tori.
Textbooks:
- Stillwell, Naive Lie Theory, Springer 2008

- Tapp, Matrix Groups for Undergraduates, Second Edition, AMS 2016

Handouts:
Worksheets:
- Complex numbers as real matrices
- Quaternions
- The geometry of space rotations
- Exercises in Lie algebras
- Symplectic matrices
- The adjoint maps
Homework:
